The Derivation of E=mc2

 

God's Equation

The Derivation of E=mc²

Perhaps the most famous equation of all time is E = mc². The equation is a direct result of the theory of special relativity, but what does it mean and how did Einstein find it? In short, the equation describes how energy and mass are related. Einstein used a brilliant thought experiment to arrive at this equation, which we will briefly review here.

First of all, let us consider a particle of light, also known as a photon. One of the interesting properties of photons is that they have momentum and yet have no mass. This was established in the 1850s by James Clerk Maxwell. However, if we recall our basic physics, we know that momentum is made up of two components: mass and velocity. How can a photon have momentum and yet not have a mass? Einstein’s great insight was that the energy of a photon must be equivalent to a quantity of mass  and hence could be related to the momentum.

Einstein’s thought experiment runs as follows. First, imagine a stationary box floating in deep space. Inside the box, a photon is emitted and travels from the left towards the right. Since the momentum of the system must be conserved, the box must recoils to the left as the photon is emitted. At some later time, the photon collides with the other side of the box, transferring all of its momentum to the box. The total momentum of the system is conserved, so the impact causes the box to stop moving.

Unfortunately, there is a problem. Since no external forces are acting on this system, the centre of mass must stay in the same location. However, the box has moved. How can the movement of the box be reconciled with the centre of mass of the system remaining fixed?

Einstein resolved this apparent contradiction by proposing that there must be a ‘mass equivalent’ to the energy of the photon. In other words, the energy of the photon must be equivalent to a mass moving from left to right in the box. Furthermore, the mass must be large enough so that the system centre of mass remains stationary.

Let us try and think about this experiment mathematically. For the momentum of our photon, we will use Maxwell’s expression for the momentum of an electromagnetic wave having a given energy. If the energy of the photon is E and the speed of light is c, then the momentum of the photon is given by:

                              

 (1.1)

The box, of mass M, will recoil slowly in the opposite direction to the photon with speed v. The momentum of the box is:

                              

 (1.2)

The photon will take a short time, Δt, to reach the other side of the box. In this time, the box will have moved a small distance, Δx. The speed of the box is therefore given by

                              

 (1.3)

By the conservation of momentum, we have

                              

 (1.4)

If the box is of length L, then the time it takes for the photon to reach the other side of the box is given by:

                              

 (1.5)

Substituting into the conservation of momentum equation (1.4) and rearranging:

                              

 (1.6)

Now suppose for the time being that the photon has some mass, which we denote by m. In this case the centre of mass of the whole system can be calculated. If the box has position x₁ and the photon has position x₂, then the centre of mass for the whole system is:

                              

 (1.7)

We require that the centre of mass of the whole system does not change. Therefore, the centre of mass at the start of the experiment must be the same as the end of the experiment. Mathematically:

                              

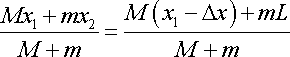
 (1.8)

The photon starts at the left of the box, i.e. x₂ = 0. So, by rearranging and simplifying the above equation, we get:

                              

 (1.9)

Substituting (1.4) into (1.9) gives:

                              

 (1.10)

Rearranging gives the final equation:

                              

So, let’s think about what this equation means. The equation suggests that a given mass can be converted into energy. But how much energy? Well, suppose we have a kilo of mass. Conversion of this mass into pure energy would result in (1kg * c²) joules of energy. Now note that c² = 8.99 * 10¹⁶ m²s^-2  so that's a WHOLE lot of energy - equivalent to 21.48 megatons of TNT!

In practice, it is not possible to convert all of the mass into energy. However, this equation led directly to the development of nuclear energy and the nuclear bomb - probably the most tangible results of special relativity.